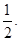Straight Lines - Solutions

CBSE Class–11 Mathematics

NCERT Solutions
Chapter - 10 Straight Lines
Exercise 10.1

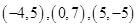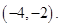1. Draw a quadrilateral in the Cartesian plane, whose vertices are and Also, find its area.

Ans. Let $A (- 4, 5), B (0, 7), C (5, - 5)$ and $D (- 4, - 2)$ be the vertices of the quadrilateral

Join the vertices A and C to obtain the diagonal AC

Area of quadrilateral ABCD = Area of triangle ABC + Area of triangle ACD....................(i)

But we have area of a triangle with vertices $(x_{1}, y_{1}), (x_{2}, y_{2})$ and $(x_{3}, y_{3})$ is

$\frac{1}{2} | x_{1} (y_{_{2}} - y_{3}) + x_{2} (y_{3} - y_{1}) + x_{3} (y_{1} - y_{2}) |$

Area of triangle ABC = $\frac{1}{2} | - 4 (7 + 5) + 0 (- 5 - 5) + 5 (5 - 7) |$

= $\frac{1}{2} | - 4 (12) + 0 + 5 (- 2) | = \frac{1}{2} | - 48 + 0 - 10 | = \frac{58}{2}$ square units

Also Area of triangle ACD = $\frac{1}{2} | - 4 (- 5 + 2) + 5 (- 2 - 5) + (- 4) (5 + 5) |$

= $\frac{1}{2} | - 4 (- 3) + 5 (- 7) + (- 4) (10) | = \frac{1}{2} | 12 - 35 - 40 | = \frac{63}{2}$ square units

From equation (i) we haveArea of quadrilateral ABCD = $\frac{58}{2} + \frac{63}{2} = \frac{121}{2}$ square units

2. The base of an equilateral triangle with side 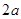lies along the axis such that the mid-point of the base is at origin. Find the vertices of the triangle.

Ans. Given: Length of side of equilateral triangle = 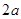. The base of triangle lies along axis and the mid-point of base is at origin so that the coordinates of vertices are 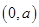and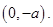

We have for an equilateral triangle line from the vertex to the mid point of the base will be perpendicular to the base.So we can say the third vertex lies on X axis (positive or negative)

Now let the third vertex be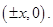

It is known that area of an equilaterl triangle = $\frac{\sqrt{3}}{4} a^{2}$ ,where a is the common length of the sides

Area of theequilaterl triangle with side 2a= $\frac{\sqrt{3}}{4} {(2 a)}^{2}$

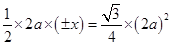
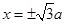
Therefore the third vertex can be $(\sqrt{3} a, 0)$ or $(- \sqrt{3} a, 0)$

The vertices of triangle are 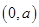, 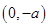and 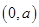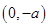$(\sqrt{3} a, 0)$ or , and $(- \sqrt{3} a, 0)$

3. Find the distance between P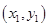 and Q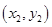 when (i) PQ is parallel to the axis (ii) PQ is parallel to the axis.

Ans. Given: P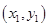 and Q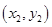 are two points.

PQ =

(i) We have equation of a line parallel to axis is X = K, a constant

PQ is parallel to axis, then $x_{2} = x_{1} \Rightarrow x_{2} - x_{1} = 0$

PQ =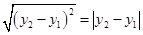

(ii) We have equation of a line parallel to axis is Y= K, a constant

PQ is parallel to axis, then

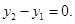
PQ =

4. Find the point on the axis, which is equidistant from the points (7, 6) and (3, 4).

Ans. Let P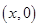 be any point on the axis which is equidistant from Q (7, 6) and R (3, 4).

We have distnce between two points $(x_{1}, y_{1})$ and $(x_{2}, y_{2})$ is $\sqrt{{(x_{2} - x_{1})}^{2} + {(y_{2} - y_{1})}^{2}}$

PQ =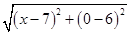

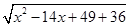
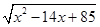
And PR =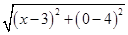

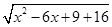
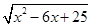
According to question, PQ = PR

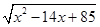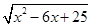
Squaring both sides, =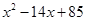

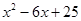
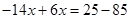
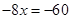
Therefore, the required point is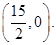

5. Find the slope of a line, which passes through the origin and the mid-point of the line segment of joining the points P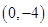 and B (8, 0).

Ans. Here, mid-point of the line segment joining P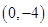 and Q (8, 0) is

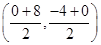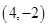= using mid point formula

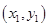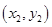We have slope of a line passing through two points and is given by $m = \frac{y_{2} - y_{1}}{x_{2} - x_{1}}$

Therefore, Slope of the line passing through the points (0, 0) and .=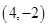

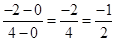
Hence slope of the required line is $\frac{- 1}{2}$

6. Without using the Pythagoras theorem, show that the points (4, 4), (3, 5) and 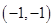are the vertices of a right angled triangle.

Ans. Let A (4, 4), B (3, 5) and C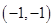 be the three vertices of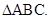

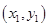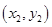We have slope of a line passing through two points and is given by $m = \frac{y_{2} - y_{1}}{x_{2} - x_{1}}$

Slope of AB =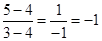

Slope of BC =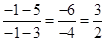

Slope of AC =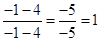

Now, Slope of AB x Slope of AC =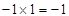

This shows that AB AC. Thus is right angled at point A.

7. Find the slope of the line, which makes an angle of 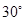with the positive direction of axis measured anticlockwise.

Ans.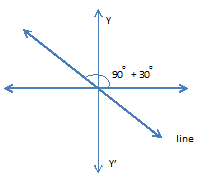

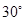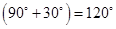If the line makes an angle of with the positive direction of axis then the line will make an angle of with the positive direction of axis.

Slope of the line =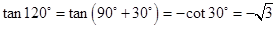

8. Find the value of 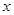for which the points 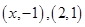and 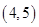are collinear.

Ans. Let A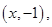 B (2, 1) and C (4, 5) be three collinear points.

Slope of AB =

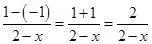
Slope of BC =

According to question, Slope of AB = Slope of BC

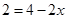
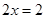
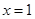
9. Without using distance formula, show that the points 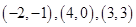and 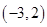are the vertices of a parallelogram.

Ans. Let A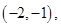 B (4, 0), C (3, 3) and D 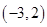be vertices of a quadrilateral ABCD.

To prove a quadrilateral is a parallelogram it is enough to show that both pairs of opposite sides are parallel

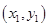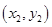We have slope of a line passing through two points and is given by $m = \frac{y_{2} - y_{1}}{x_{2} - x_{1}}$

Slope of AB = 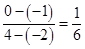Slope of BC =

Slope of DC = 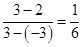Slope of AD =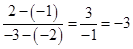

Here Slope of AB = Slope of DC

AB DC

And Slope of BC = Slope of AD

BC AD

Therefore, ABCD is a parallelogram.

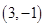10. Find the angle between the axis and the line joining the points and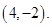

Ans. Let A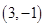 and B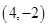 be two points. Let $θ$ be the angle which AB makes with positive direction of axis.

Slope of AB = 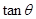.................(i)

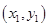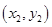We have slope of a line passing through two points and is given by $m = \frac{y_{2} - y_{1}}{x_{2} - x_{1}}$

Slope of AB = 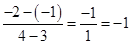....................(ii)

From (i) and (ii) we get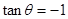

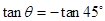
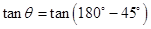
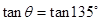
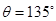
11. The slope of a line is double of the slope of the another line. If tangent of the angle between them is 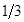find the slopes of the lines.

Ans. Given: 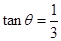. Let the slopes of two lines be and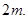

We know that if $θ$ is the acute angle between two lines with slopes $m_{1}$ and $m_{2}$ respectively

then $\tan θ = | \frac{m_{2} - m_{1}}{1 + m_{1} m_{2}} |$

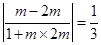
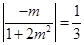
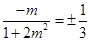
Taking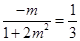

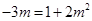
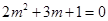
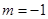$(m + 1) (2 m + 1) = 0$

and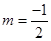

When 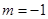the slopes of the lines are -1 and -2

when 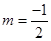the slopes of the lines are $\frac{- 1}{2}$ and -1

Taking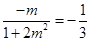

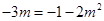
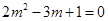
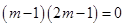
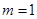and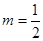

When m=1 the slopes of the lines are 1 and 2

when 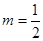the slopes of the lines are $\frac{1}{2}$ and 1

Therefore, the slopes of lines are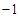and $- 2$ or 1 and 2 or 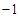and 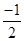or 1 and